Martingale With Respect To Filtration . — property (a), together with the previous additive property, means that the collection of martingales with respect to a. learn about conditional expectations, filtration and martingales in stochastic processes. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. Learn the basic theory, assumptions, and. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse.
from www.numerade.com
does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? — property (a), together with the previous additive property, means that the collection of martingales with respect to a. given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. learn about conditional expectations, filtration and martingales in stochastic processes. Learn the basic theory, assumptions, and. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t.
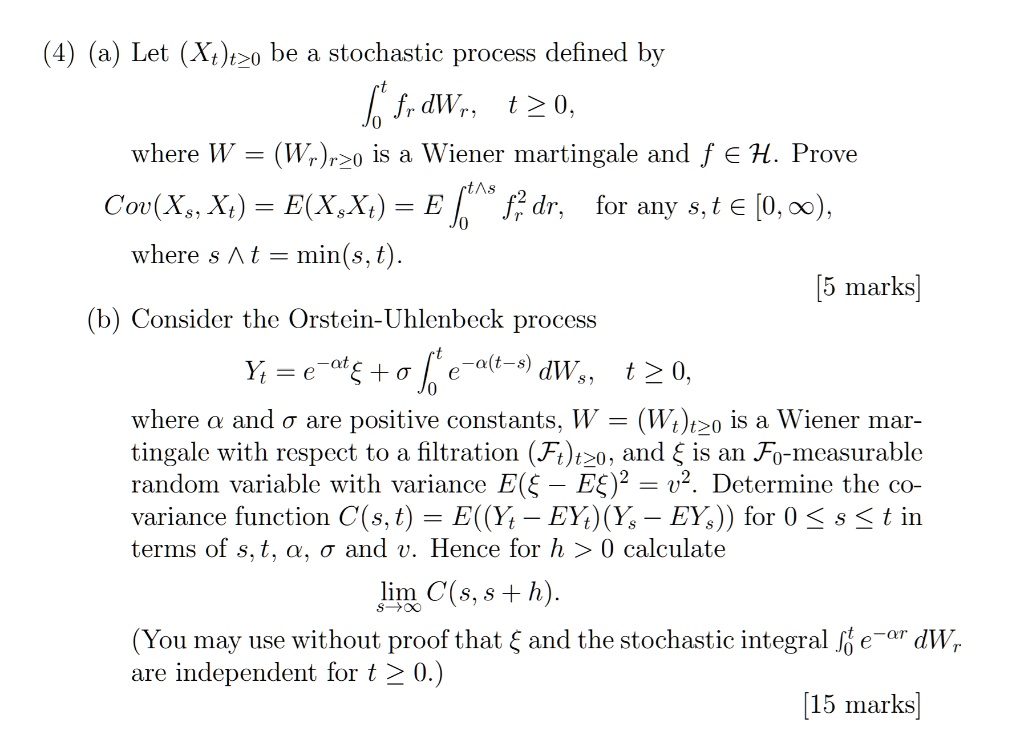
SOLVED Let (Xt)t≥0 be a stochastic process defined by Jf,aWrs t > 0
Martingale With Respect To Filtration learn about conditional expectations, filtration and martingales in stochastic processes. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. learn about conditional expectations, filtration and martingales in stochastic processes. Learn the basic theory, assumptions, and. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t.
From www.researchgate.net
(PDF) Martingale Representation in the Enlargement of the Filtration Martingale With Respect To Filtration learn about conditional expectations, filtration and martingales in stochastic processes. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. does filtration discretize the time space of a stochastic process so that we. Martingale With Respect To Filtration.
From www.numerade.com
SOLVED State the optional stopping theorem for martingales Let x Martingale With Respect To Filtration Learn the basic theory, assumptions, and. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. does filtration discretize the time space of a stochastic process so. Martingale With Respect To Filtration.
From www.numerade.com
SOLVEDExercise (Riskneutral probabilities make the stock price a Martingale With Respect To Filtration learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. Learn the basic theory, assumptions, and. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? given a. Martingale With Respect To Filtration.
From www.researchgate.net
(PDF) A martingale bound for the entropy associated with a trimmed Martingale With Respect To Filtration learn about conditional expectations, filtration and martingales in stochastic processes. Learn the basic theory, assumptions, and. given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. learn the basics of martingale theory, including submartingales, stopping. Martingale With Respect To Filtration.
From www.researchgate.net
(PDF) Martingale representation property in progressively enlarged Martingale With Respect To Filtration given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. — property (a), together with the previous additive property, means that the collection of. Martingale With Respect To Filtration.
From www.researchgate.net
(PDF) On the Martingale Representation with Respect to the super Martingale With Respect To Filtration learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? Learn the basic theory, assumptions, and. learn about conditional expectations, filtration and martingales in stochastic processes. learn the definition, properties and examples. Martingale With Respect To Filtration.
From slideplayer.com
5.4 Fundamental Theorems of Asset Pricing 報告者:何俊儒. ppt download Martingale With Respect To Filtration learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? — property (a), together with the previous additive property, means that the collection of martingales with respect to a. given a filtration. Martingale With Respect To Filtration.
From gainium.io
DCA bot basics Martingale With Respect To Filtration learn about conditional expectations, filtration and martingales in stochastic processes. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. learn. Martingale With Respect To Filtration.
From slideplayer.com
Matingales and Martingale Reprensentations ppt download Martingale With Respect To Filtration learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? — property (a), together with the previous additive property, means that the collection of martingales with respect to a. given a filtration. Martingale With Respect To Filtration.
From slideplayer.com
Matingales and Martingale Reprensentations ppt download Martingale With Respect To Filtration does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? learn about conditional expectations, filtration and martingales in stochastic processes. given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to. — a martingale is a stochastic process that satisfies. Martingale With Respect To Filtration.
From theculture.sg
Introduction to Martingales The Culture SG Martingale With Respect To Filtration learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. does filtration discretize the time space of a stochastic. Martingale With Respect To Filtration.
From www.numerade.com
SOLVED (A useful criterion for martingales) Given a probability space Martingale With Respect To Filtration — property (a), together with the previous additive property, means that the collection of martingales with respect to a. does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. learn the basics. Martingale With Respect To Filtration.
From www.slideserve.com
PPT 4.4 ItoDoeblin Formula(part2) PowerPoint Presentation, free Martingale With Respect To Filtration — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. Learn the basic theory, assumptions, and. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. learn about conditional expectations, filtration and. Martingale With Respect To Filtration.
From www.researchgate.net
(PDF) A martingale approach to reliability theory On the role of Martingale With Respect To Filtration — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and reverse. given a filtration \((\mathcal{f}_t)_{t \leq t}\) and a random variable \(z\) which is measurable with respect to.. Martingale With Respect To Filtration.
From math.stackexchange.com
probability theory Preservation of the local martingale property Martingale With Respect To Filtration learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. learn about conditional expectations, filtration and martingales in stochastic processes. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. learn the basics of martingale theory, including submartingales, stopping times, doob decomposition, and. Martingale With Respect To Filtration.
From www.slideserve.com
PPT Joint Advanced Student School 2004 Complexity Analysis of String Martingale With Respect To Filtration learn the definition, properties and examples of martingales, submartingales and supermartingales in probability theory. — property (a), together with the previous additive property, means that the collection of martingales with respect to a. learn about conditional expectations, filtration and martingales in stochastic processes. does filtration discretize the time space of a stochastic process so that we. Martingale With Respect To Filtration.
From electrofx.com
Low Risk Martingale To be Treated With Maximum Respect! Martingale With Respect To Filtration does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? Learn the basic theory, assumptions, and. learn about conditional expectations, filtration and martingales in stochastic processes. — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈. Martingale With Respect To Filtration.
From www.researchgate.net
(PDF) Nonextremal martingale with Brownian filtration Martingale With Respect To Filtration does filtration discretize the time space of a stochastic process so that we can analyze the process as a martingale? — a martingale is a stochastic process that satisfies the condition e(xt | fs) = xs for all s, t ∈ t with s ≤ t. learn about conditional expectations, filtration and martingales in stochastic processes. . Martingale With Respect To Filtration.